Journal of Optics, 12th of June 2017
This article belongs to the flourishing research field of spatial nonlinearity studies in waveguides, branch of nonlinear optics where the spatial profiles of the electromagnetic fields are taken into account in order to accurately describe the nonlinear phenomena. At the Fresnel Institute, this research topic has started in 2008 with a first work dealing with the extension of the well-known Townes soliton.
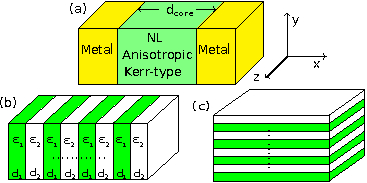
In their new article, Gilles Renversez and his PhD student Mahmoud Elsawy from the Institut Fresnel (CNRS/AMU/ECM) have build two distinct models to investigate the transverse magnetic stationary solutions propagating in one-dimensional anisotropic nonlinear plasmonic structures made from a Kerr-type nonlinear metamaterial core embedded between two semi-infinite metal claddings. The first model is semi-analytical, in which we assume that the anisotropic nonlinearity depends only on the transverse component of the electric field and that the nonlinear refractive index modification is small compared to the linear one. This method allows us to derive analytically the field profiles and nonlinear dispersion relations in terms of the Jacobi elliptical functions. The second model is fully numerical and is based on the finite element method in which all the components of the electric field are considered in the Kerr-type nonlinearity, with no presumptions as to the nonlinear refractive index change. Our finite-element-based model is valid beyond the weak nonlinearity regime and generalizes the well-known single-component fixed power algorithm that is usually used. Examples of the main cases are investigated, including those with strong spatial nonlinear effects at low power. Loss issues are reduced through the use of a gain medium in the nonlinear metamaterial core. Using anisotropic nonlinear FDTD simulations, some results for the properties of the main solution are also provided. These results should allow to fabricate nonlinear waveguides with fairly reduced lengths compared to the usual ones due to the large increase of the effective nonlinearity even at low powers.
References:
Mahmoud M. R. Elsawy and Gilles Renversez, "Study of plasmonic slot waveguides with a nonlinear metamaterial core: semi-analytical and numerical methods" Téléchargeable gratuitement pendant dix jours à partir du 12 juin.
Published 9 June 2017 - Journal of Optics, Volume 19, Number 7
See also one application of these new methods in our very recent article:
Mahmoud M. R. Elsawy and Gilles Renversez, "Spatial Nonlinearity in Anisotropic Metamaterial Plasmonic Slot Waveguides" published also in June 2017 in the Plasmonics journal
Contact:
Institut Fresnel - CNRS UMR 7249, Aix-Marseille Université, 13013, Marseille, France
Gilles RENVERSEZ, Professor at Aix-Marseille University and member of ATHENA group.
gilles.renversez@fresnel.fr - Phone : +33 4 91 28 89 85


 ResearchGate
ResearchGate Flux RSS
Flux RSS